

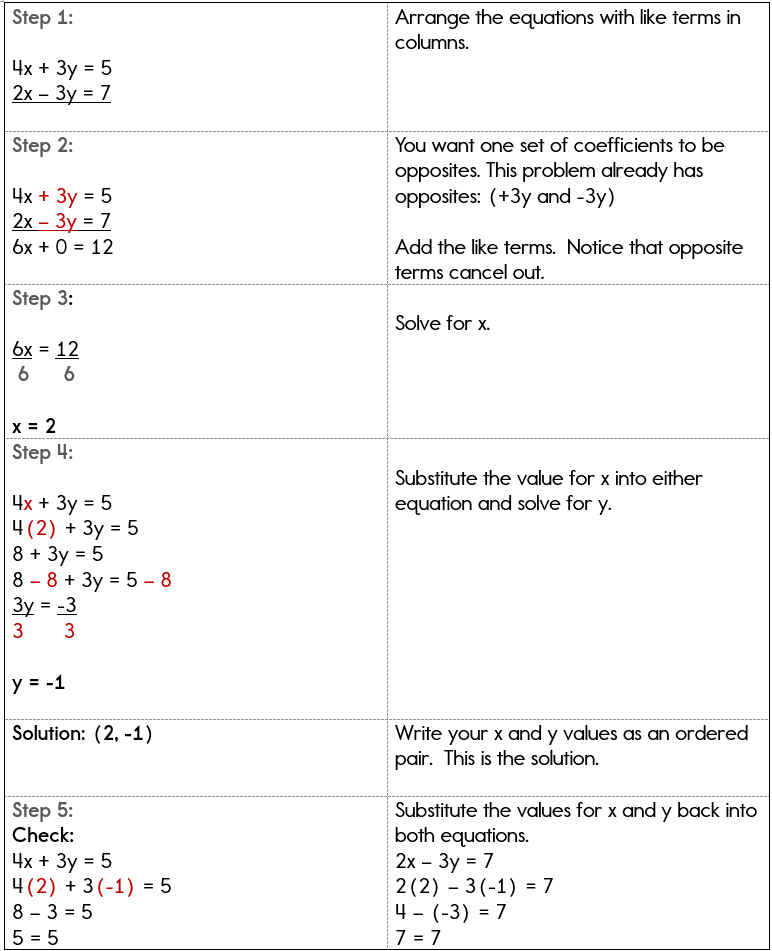
f + s = 164 85 + s = 164 s = 79 The second test score is 79. SolveThen substitute 85 for f in one of the original equations to solve for s. First use elimination on the last two equations to solve for f.f s = 6 f + s = 164 2f = 170 f = 85 The first test score is 85. PlanWrite the system of equations from the information given.į + s + t = 256 f s = 6 f + s = 164The total of the scores is 256.The difference between the 1st and 2nd is 6 points.The total before taking the third test is the sum of the first and second tests. Try solving the problemLet f = Courtneys score on the first testLet s = Courtneys score on the second testLet t = Courtneys score on the third test. Solving these systems is very similar to solving systems of equations in two variables. What were Courtneys test scores on the three tests?ĮxploreProblems like this one can be solved using a system of equations in three variables. His total score before taking the third test was 164 points. His score on the first test exceeds his score on the second by 6 points. It is very helpful to neatly organize yourwork on your paper in the following manner.(x, y, z) X 3(2) + 6(5) = 21x 6 + 30 = 21 x + 24 = 21x = 3ĬHECK the solution in ALL 3 of the original equations. Substitute the value of the variables from the system of two equations in one of the ORIGINAL equations with three variables. Solve the system for the two remaining variables. Write the resulting equations in two variables together as a system of equations. X 3y + 6z = 21 x 3y + 6z = 213x + 2y 5z = 30 2x 5y + 2z = 6Įliminate THE SAME variable in each of the two smaller systems.Īny variable will work, but sometimes one may be a bit easier to eliminate. Rewrite the system as two smaller systems, each containing two of the three equations. Use elimination to solve the following system of equations. Yes, it is a solution to the system because it is a solution to all 3 equations. The solution to a system of three linear equations in three variables is an ordered triple. Solving Systems of Three Linear Equations in Three VariablesThe Elimination Method


 0 kommentar(er)
0 kommentar(er)
